^Resolving power of optical instruments

^Resolving power of optical instruments

Suppose a convex lens is used to form the image of an object. Consider a parallel beam of light falling on it. If the lens is well corrected for aberrations, then geometrical optics tells us that the beam will get focused to a point, producing a sharp image point. However, because of diffraction, the beam instead of getting focused to a point gets focused to a spot of finite area in the form of alternate bright & dark concentric circles around a central bright disc as shown in figure. This spot is called the diffraction pattern.

A detailed analysis shows that the radius of the central bright region is approximately given by,

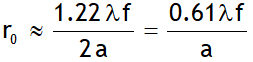
Thus the conclusion is a parallel beam of light incident on a convex lens gets focused to a spot of radius, 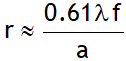  because of diffraction effects.  Where f is the focal length of the lens and 2 a (= d) is the diameter of the circular aperture or the diameter of the lens.